clinicspot.blogg.se

Since the triangles ABE and CDE are similar, then these are ratios of corresponding sides.

If we label as r the ratio r = AB/CD, then the diagonals are divided by this ratio AE/CE = BE/DE = r. Given a trapezoid ABCD with parallel sides AB and CD, let E be the intersection of the diagonals AC and BD. Thus by AA, the triangles ABE and CDE are similar. By the diagonals are transversals, so the marked angles are equal: angle BAE = angle DCE and angle ABD = angle CDE. Construct point E as the intersection of the diagonals AC and BD. In the event we wish to distinguish trapezoids with exactly two parallel sides, we will call such trapezoids strict trapezoids. In Math 444 the official definition of a trapezoid is the Inclusive Definition. However, it is important to have agreement in a math class on the definition used in the class. It is possible to function perfectly well with either definition. This fits best with the nature of twentieth-century mathematics. The advantage of the inclusive definition is that any theorem proved for trapezoids is automatically a theorem about parallelograms. This seems to have been most important in earlier times. The advantage of the first definition is that it allows a verbal distinction between parallelograms and other quadrilaterals with some parallel sides.

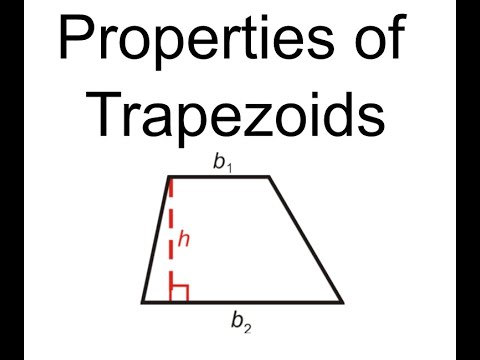
Trapezoids and under the first, they are not. The difference is that under the second definition parallelograms are However, most mathematicians would probably define the concept with theĪ quadrilateral having at least two sides parallel is called a Two and only two sides parallel is called a trapezoid. In B&B and the handout from Jacobs you got the Exclusive Definition. Believe it or not, there is no general agreement on the definition of a trapezoid.